Unlock the Power of Compounding with Our Free Excel Compound Interest Calculator. Download Now!
There’s a popular quote attributed to Albert Einstein: “Compound interest is the eighth wonder of the world. He who understands it, earns it; he who doesn’t, pays it.” Whether or not Einstein actually said this, the truth it encapsulates is undeniable. Compound interest can either work as your best ally or your worst enemy. Understanding it is often the defining factor between financial prosperity and financial distress, and that’s where our Compound Interest Calculator Excel Template comes in.
I. Decoding Compound Interest
A. Definition
At its core, compound interest is the interest calculated on a loan or deposit based on the initial principal and the accumulated interest from previous periods. It enables your savings to accelerate over time, as compared to simple interest, which only grows at a steady, linear pace.
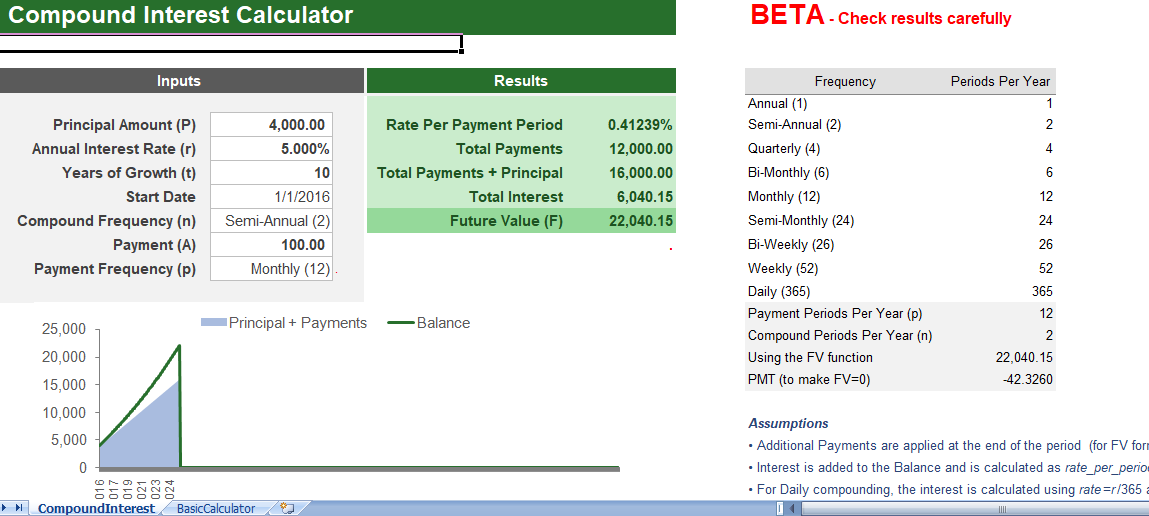
This spreadsheet is designed as an educational tool – to help you show how compound interest works for both savings and loans. The table is based on the payment frequency and shows the amount of interest adds in each period. The graph compares the total principal and payments to the balance over time.
Compound Interest Formula
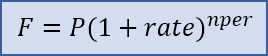
The basic compound interest formula for calculating a future value is F = P*(1+rate)^nper where
- F = future accumulated value
- P = principal (starting) amount
- rate interest rate per compounding period
- nper =total number of compounding periods
Formula for Compounding Yearly, Monthly, Weekly
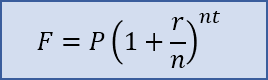
- P = the principal amount
- r = the nominal annual interest rate in decimal form.
- n = the number of compound periods per year
- t = the time in years
Compound Interest formula Calculator
This is the basic formula where rate = r/n and nper = n*t. Although the math can handle a decimal value for nper, it should usually be a whole number. Traditional amortize loans use the same formulas as define for savings, except that the loan amount represents as a negative value for the starting principal, P. Payment amounts (A) are the positive values. In Excel we can use the FV function again. The formulas show how the FV function relates to the standard formula. Note that for now we aren’t including a principal amount.
B. Importance of Compound Interest
Calculating compound interest is critically important for several reasons:
- Financial Planning: It serves as a vital tool for financial planning, enabling you to project how your investments or savings will grow over time.
- Informed Decisions: It aids in making informed decisions when it comes to loans, investments, and savings.
- Comparative Analysis: It enables you to compare different loan or investment options on a similar scale.
- Optimize Growth: It assists in understanding and optimizing your financial growth, whether as an individual or a business.
II. Objective and Use Cases of Our Calculator
The primary objective of our Compound Interest Calculator Excel Template is to streamline the often complex process of calculating and understanding compound interest. This tool is an excellent asset for anyone, from individuals trying to estimate their savings growth or debt repayment schedules, to financial advisors and businesses aiming to calculate investment returns or loan schedules.
III. Why You Should Use Our Calculator
Our Compound Interest Calculator is not just a tool to crunch numbers. It’s an indispensable financial planning asset offering several benefits:
- Ease of Use: Simply enter your principal amount, interest rate, growth years, start date, compound frequency, payments, and payment frequency to instantly calculate the results.
- Visualized Charts: The template includes visualized charts showing the principal, payments, and balance over time, enabling a better understanding of your financial trajectory.
- Free and Accessible: The calculator is absolutely free. You can use it multiple times to strategize different scenarios.
- Time-saving: It saves you from doing manual calculations, letting the template do all the heavy lifting.
Understanding compound interest is key to achieving your financial goals. With our Compound Interest Calculator, you’re not just gaining a tool for calculations, but a window into your financial future.
IV. Envisioning the Power of Compound Interest
With our Compound Interest Calculator Excel Template, you gain much more than a mere number-crunching tool. It brings the power of compound interest to life, offering a clear window into your financial future.
A. Project Your Financial Future
The calculator enables you to project various financial scenarios and trajectories based on your inputs. You can observe how your investments or savings could potentially grow over time or see how changing variables can affect your loan repayment schedule. It’s like having a crystal ball that lets you peek into your financial future!
B. Smart Financial Planning
Our Compound Interest Calculator acts as a compass for your financial journey. By projecting your investment or loan schedules, you can effectively plan and strategize to optimize growth or pay off debts. It helps you understand how different rates, frequencies, and timeframes could influence your financial landscape. This knowledge equips you with the foresight needed for smarter financial planning.
V. Getting the Most Out of Our Compound Interest Calculator
A. Test Different Scenarios
Don’t just calculate once! Play around with different scenarios to see how slight tweaks in the variables can lead to significant changes in the outcome. Experiment with different interest rates, different frequencies of compounding, or different investment durations. By doing so, you gain valuable insights into how these variables interplay in the grand scheme of your financial growth.
B. Leverage the Visualized Charts
One of the unique features of our calculator is the set of visualized charts it generates based on your inputs. These charts offer a visual representation of your principal, payments, and balance over time. They serve to enhance your understanding of how compound interest works and help you visualize your growth or repayment trajectory more tangibly.
VI. Conclusion
In the world of finance, knowledge is power, and understanding compound interest is a significant part of that knowledge. With our free, user-friendly, and powerful Compound Interest Calculator Excel Template, you are well-equipped to take control of your financial future. It’s not just about calculating compound interest; it’s about unlocking the power of compounding to build a secure and prosperous financial future.